Question 128

If the external bisector of the vertical angle $$\angle$$ A of the $$\triangle$$ ABC is parallel to the base BC, then the $$\triangle$$ ABC is a/an

Solution

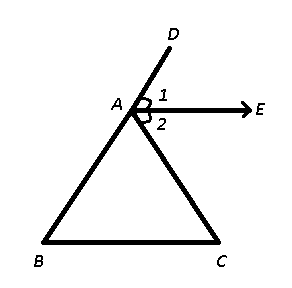
AE is the bisector of the exterior $$\angle$$ DAC of the $$\triangle$$ ABC and AE || BC

Now, $$\angle$$ $$1$$ = $$\angle$$ $$2$$ (given)

Also, $$\angle$$ $$B$$ = $$\angle$$ $$1$$ (Corresponding angle)

and $$\angle$$ $$C$$ = $$\angle$$ $$2$$ (Alternate angle)

=> $$\angle$$ $$B$$ = $$\angle$$ $$C$$

=> AB = AC

So, $$\triangle$$ ABC is an isosceles triangle.

=> Ans - (B)

View More Questions From Geometry

Create a FREE account and get: