A fruit seller has a stock of mangoes, bananas and apples with at least one fruit of each type. At the beginning of a day, the number of mangoes make up 40% of his stock. That day, he sells half of the mangoes, 96 bananas and 40% of the apples. At the end of the day, he ends up selling 50% of the fruits. The smallest possible total number of fruits in the stock at the beginning of the day is

(Note: This was a repeated question that appeared in both CAT 2023 and CAT 2024)

Let us assume the initial stock of all the fruits is S.

Let us take we have 'b' and 'a' mangoes initially.

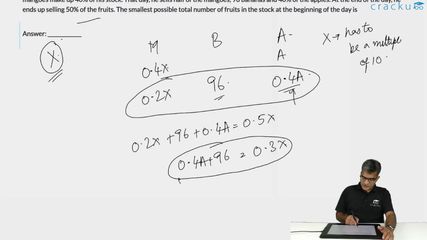
Stock of Mangoes = 40% of S = 2S/5

The total number of fruits sold are Mangoes Sold + Apples Sold + Bananas Sold

= 2S/10 + 96 + 4a/10 = S/2 (Given)

=> S/5 + 96 + 2a/5 = S/2

=> S = $$\dfrac{\left(4a+960\right)}{3}$$

=> $$\dfrac{4a}{3}+320$$

'a' has to be a multiple of 3 for the above term to be an integer.

But 'a' has to be a multiple of 5 for 4a/10 to be an integer.

=> The smallest value of 'a' satisfying both conditions is 15.

=> $$\dfrac{4a}{3}+320=\dfrac{4\left(15\right)}{3}+320$$ = 340

Therefore, 340 is the correct answer.