An isosceles triangle ABC has sides AB = AC. If side BA is produced to D in such a manner that AC = AD. Then what will be the measure of angle BCD?

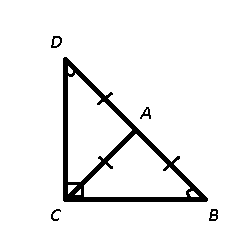
Given : ABC is an isosceles triangle and AB = AC = AD

To find : $$\angle$$ BCD = ?

Solution : $$\because$$ AB = AC, => $$\angle$$ ACB = $$\angle$$ ABC = $$\theta$$

Similarly, $$\angle$$ ACD = $$\angle$$ ADC = $$\theta$$

Now, in $$\triangle$$ BCD, 

=> $$\angle$$ ABC + ($$\angle$$ ACB + $$\angle$$ ACD) + $$\angle$$ ADC = $$180^\circ$$

=> $$\theta+\theta+\theta+\theta=180^\circ$$

=> $$\theta=\frac{180^\circ}{4}=45^\circ$$

$$\therefore$$ $$\angle$$ BCD = $$\theta+\theta=45^\circ+45^\circ=90^\circ=\frac{\pi}{2}$$

=> Ans - (D)