Question 77

Let ABC be a right-angled triangle with hypotenuse BC of length 20 cm. If AP is perpendicular on BC, then the maximum possible length of AP, in cm, is

Name: Let ABC be a right-angled triangle with hypotenuse BC of length 20 cm. If AP is perpendicular on BC, then the maximum possible length of AP, in cm, is
Uploaded: May 3, 2022, 4:57 p.m.
Duration: 4 min 34 s
Description: Let ABC be a right-angled triangle with hypotenuse BC of length 20 cm. If AP is perpendicular on BC, then the maximum possible length of AP, in cm, is

Solution

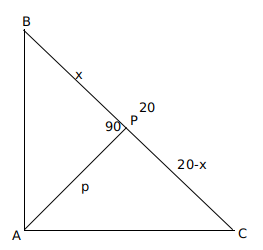
Let p be the length of AP.

It is given that $$\angle\ BAC\ =90\ $$ and $$\angle\ APC\ =90\ $$

Let $$\angle\ ABC\ =\theta\ $$, then $$\angle\ BAP\ =90-\theta\ $$ and $$\angle\ BCA\ =90-\theta\ $$

So $$\angle\ PAC\ =\theta\ $$

Triangles BPA and APC are similar

$$p^2=x\left(20-x\right)$$

We have to maximize the value of p, which will be maximum when x=20-x

x=10

Share on Whatsapp Share on Whatsapp

Boost your CAT prep: Take All-India 3 free CAT mock tests with Video solutions

Video Solution

Click on the Email ☝️ to Watch the Video Solution

Create a FREE account and get:

All Quant CAT complete Formulas and shortcuts PDF
38+ CAT previous year papers with video solutions PDF
5000+ Topic-wise Previous year CAT Solved Questions for Free

CAT Quant Questions | CAT Quantitative Ability

CAT Profit, Loss and Interest Questions CAT Remainders Questions CAT Geometry Questions CAT Averages Mixtures Alligations Questions CAT Averages Questions

CAT DILR Questions | LRDI Questions For CAT

CAT Data Interpretation Questions CAT Selection With Condition Questions CAT Charts Questions CAT DI Miscellaneous Questions CAT Special Charts Questions

CAT Verbal Ability Questions | VARC Questions For CAT

CAT Odd One Out Questions CAT Reading Comprehension Questions CAT Vocabulary Questions CAT Para Summary Questions CAT Critical Reasoning Questions

Let ABC be a right-angled triangle with hypotenuse BC of length 20 cm. If AP is perpendicular on BC, then the maximum possible length of AP, in cm, is

Video Solution

CAT Quant Questions | CAT Quantitative Ability

CAT DILR Questions | LRDI Questions For CAT

CAT Verbal Ability Questions | VARC Questions For CAT

Also Read

Related Formulas With Tests

CAT 2019 QA Slot 2 Question

Let ABC be a right-angled triangle with hypotenuse BC of length 20 cm. If AP is perpendicular on BC, then the maximum possible length of AP, in cm, is

Video Solution

CAT Quant Questions | CAT Quantitative Ability

CAT DILR Questions | LRDI Questions For CAT

CAT Verbal Ability Questions | VARC Questions For CAT

Also Read

Free CAT Quant Questions

Related Formulas With Tests

Login to your Cracku account

Hello! 👋

Ready to Unlock Your Success?

Please Enter Your OTP

Please enter the following details

Create a free Cracku account

Get Started with 5000+ Free CAT Questions & Mock Tests with Detailed Solutions!