Question 5

If the length of the transverse tangent is 8 cm, then calculate the centre distance (in cm) of the two circles with radii 7 cm and 3 cm.

Solution

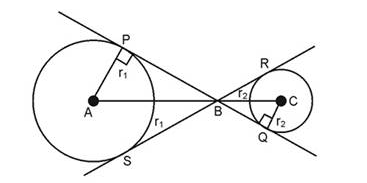
PQ and RS are traverse common tangents

Let the distance between centres AC = $$d$$ cm

Then, length of traverse common tangent is given by = $$(PQ)^2=(RS)^2=d^2-(r_1+r_2)^2$$

=> $$(8)^2=d^2-(7+3)^2$$

=> $$d^2=100+64=164$$

=> $$d=\sqrt{164}$$ cm

=> Ans - (C)

Share on Whatsapp Share on Whatsapp

Create a FREE account and get: