Please enter the following details
Free Daily Targets & Mocks
Study Material & Formulas PDFs
1 on 1 Mentorship
Question 65
In a square PQRS, A and B are two points on PS and SR such that PA =2AS, and RB = 2BS If PQ = 6, the area of the triangle ABQ is (in sq. cm)
Solution
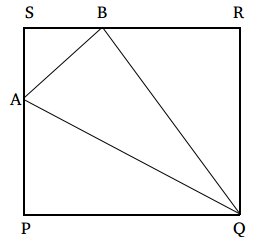
PQ = QR = RS = SP = 6 cm
PA = 2AS and PA + AS = 6cm
=> PA = 4 cm and AS = 2 cm
Similarly, RB = 2BS and RB + BS = 6 cm
=> RB = 4 cm and BS = 2cm
Area of △ ABQ = area of PQRS - area of △APQ - area of △RBQ - area of △ASB
= (36 - 12 - 12 - 2) sq. cm
= 10 sq. cm
Hence, option C is the correct answer.
Create a FREE account and get:
- All Quant Formulas and shortcuts PDF
- 15 XAT previous papers with solutions PDF
- XAT Trial Classes for FREE
XAT Quant Questions | XAT Quantitative Ability
XAT Venn Diagrams QuestionsXAT Data Sufficiency QuestionsXAT Time, Distance and Work QuestionsXAT Progressions and Series QuestionsXAT Averages, Ratio and Proportion Questions
XAT DILR Questions | LRDI Questions For XAT
XAT Games and Tournamnents QuestionsXAT Puzzles QuestionsXAT Logical Reasoning QuestionsXAT Data Interpretation QuestionsXAT Scheduling Questions
