In a school, the number of students in each class, from Class I to X, in that order, are in an arithmetic progression. The total number of students from Class I to V is twice the total number of students from Class VI to X.

If the total number of students from Class I to IV is 462, how many students are there in Class VI?

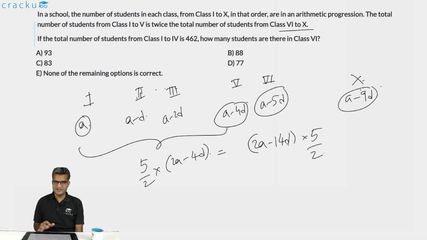
The number of students from 1 to 10 is in AP. Let's assume the series as follows:

a, a+d, a+2d, a+3d, a+4d, a+5d, a+6d, a+7d, a+8d, a+9d.

Given that the sum of the number of students in the first four classes is 462.

So, a +a+d+ a+2d+ a+3d=4a+6d=462 => 2a +3d=231--->1

It is also given that the total number of students from Class I to V is twice the total number of students from Class VI to X.

So, 5a+10d = 2*(5a+35d).

5a+10d=10a+70d

5a+60d=0 --> 2

Solving equations 1 and 2.

Multiply equation 1 with 20.

We get 40a+60d=4620--->3. Subtract equation 2 from equation 3.

We get 35a=4620

=> a=132.

Substitute a value in equation 2. We get 60d=-660=>d=-11.

Now, we have to calculate the number of students in class VI.

That is a+5d=132-5*11=77.