XAT 2022 Question Paper - Quant
For the following questions answer them individually
A supplier receives orders from 5 different buyers. Each buyer places their order only on a Monday. The first buyer places the order after every 2 weeks, the second buyer, after every 6 weeks, the third buyer, after every 8 weeks, the fourth buyer, every 4 weeks, and the fifth buyer, after every 3 weeks. It is known that on January 1st, which was a Monday, each of these five buyers placed an order with the supplier.
On how many occasions, in the same year, will these buyers place their orders together excluding the order placed on January 1st?
Some members of a social service organization in Kolkata decide to prepare 2400 laddoos to gift to children in various orphanages and slums in the city, during Durga puja. The plan is that each of them makes the same number of laddoos. However, on the laddoo-making day, ten members are absent, thus each remaining member makes 12 laddoos more than earlier decided.
How many members actually make the laddoos?
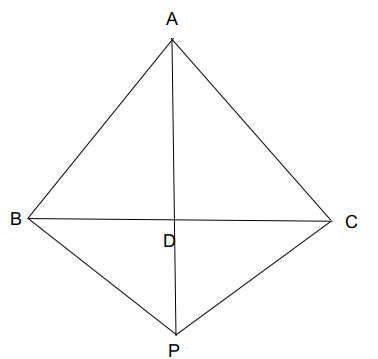
Ramesh and Reena are playing with triangle ABC. Ramesh draws a line that bisects $$\angle BAC$$; this line cuts BC at D. Reena then extends AD to a point P. In response, Ramesh joins B and P. Reena then announces that BD bisects $$\angle PBA$$, hat a surprise! Together, Ramesh and Reena find that BD= 6 cm, AC= 9 cm, DC= 5 cm, BP=8 cm, and DP = 5 cm.
How long is AP?
Sheela purchases two varieties of apples - A and B - for a total of Rupees 2800. The weights in kg of A and B purchased by Sheela are in the ratio 5 : 8 but the cost per kg of A is 20% more than that of B. Sheela sells A and B with profits of 15% and 10% respectively.
What is the overall profit in Rupees?
A marble is dropped from a height of 3 metres onto the ground. After hitting the ground, it bounces and reaches 80% of the height from which it was dropped. This repeats multiple times. Each time it bounces, the marble reaches 80% of the height previously reached. Eventually, the marble comes to rest on the ground.
What is the maximum distance that the marble travels from the time it was dropped until it comes to rest?
The sum of the cubes of two numbers is 128, while the sum of the reciprocals of their cubes is 2.
What is the product of the squares of the numbers?
Nadeem’s age is a two-digit number X, squaring which yields a three-digit number,whose last digit is Y. Consider the statements below:
Statement I: Y is a prime number
Statement II: Y is one-third of X
To determine Nadeem’s age uniquely:
A tall tower has its base at point K. Three points A, B and C are located at distances of 4 metres, 8 metres and 16 metres respectively from K. The angles of elevation of the top of the tower from A and C are complementary.
What is the angle of elevation (in degrees) of the tower’s top from B?
Read the following scenario and answer the THREE questions that follow.
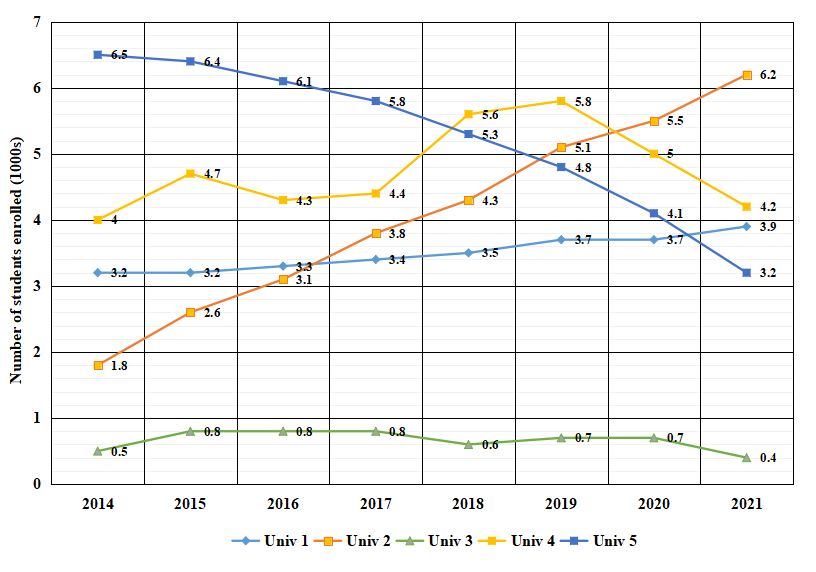
The enrolment of students (in 1000s) at each of the five universities named — MPU, JSU, LTU, PKU and TRU — during each of the eight years from 2014 to 2021 is represented in the following chart. The names of these universities are not shown in the chart, Stead they are labelled Unit 1, Unit 2, Unit 3, Unit 4 and Unit 5.
However, these four pieces of information are available:
W: The magnitudes of TRU's and MPU's net change in enrolment between 2014 and 2021 are the closest among any two universities.
X: LTU had the same enrolment in consecutive years at least twice between 2014 and 2021.
Y: The increase in JSU's enrolment from 2015 to 2019 is about 50% of TRU's total enrolment in 2020.
Z: The enrolment in one of LTU and PKU had a steady decline between 2014 and 2021, while the enrolment in the other had no decline between any two consecutive years in the same period.
Which amongst the pieces of information mentioned below, if removed, will notprevent us from uniquely identifying the five universities?
Read the following scenario and answer the THREE questions that follow.
A pencil maker ships pencils in boxes of size 50, 100 and 200. Due to packaging issues, some pencils break. About the 20 boxes he has supplied to a shop, the following information is available:
* Box no. 1 through 6 have 50 pencils, Box no. 7 through 16 have 100 pencils and Box no. 17 through 20 have 200 pencils.
* No box has less than 5% or more than 20% broken pencils.
Following is the frequency table of the number of broken pencils for the twenty boxes:
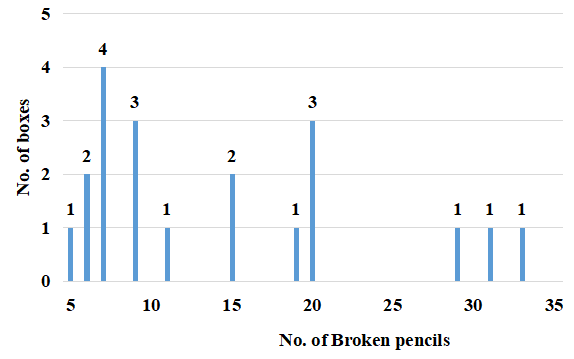
Which of the following can possibly be the sequence of the number of brokenpencils in Boxes 7-16?
Suppose that additionally it is known that the number of broken pencils in Boxes 17-20 are in increasing order. Which among the following additional information, if true, is not sufficient to uniquely know the number of defective pencils in each of the boxes numbered 17-20?
Read the following scenario and answer the THREE questions that follow.
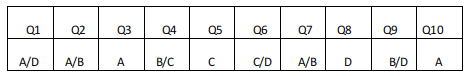
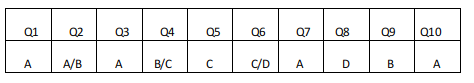
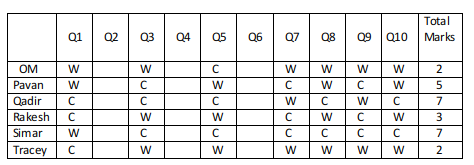
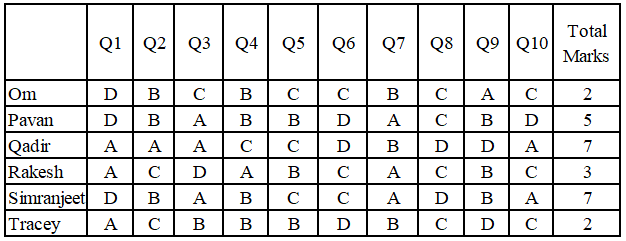
An examination had ten multiple choice questions; labelled Q1 to Q10 respectively. Each question had four answer options — A, B, C and D — of which one and only one was the correct answer. For each correct answer, the candidate obtained 1 mark. There were no negative marks for wrong answers. The answers chosen by six candidates named Om, Pavan, Qadir, Rakesh, Simranjeet and Tracey to each of the ten questions and the total marks obtained by each of them are shown in the table.
For the following questions answer them individually
Shireen draws a circle in her courtyard. She then measures the circle’s circumference and its diameter with her measuring tape and records them as two integers, A and B respectively. She finds that A and B are co-primes, that is, their greatest common divisor is 1. She also finds their ratio, A:B, to be: 3.141614161416… (repeating endlessly).
What is A - B ?
Read the following scenario and answer the THREE questions that follow.

The given candlestick chart depicts the prices of a particular stock over 10 consecutive days. A candlestick comprises of a rectangular box pieced by a line. The top and bottom ends of the line respectively indicate the maximum and minimum prices of the stock on that day, while the horizontal edges of the rectangle correspond to the stock's opening and closing prices. If the rectangle is white, the opening price is lower than the closing price, but if the rectangle is black, then it is the other way around.
Using the above information, answer the questions that follow:
Which day saw the maximum percentage increase in the stock price at closing from the opening?
What is the highest magnitude of change over two consecutive days (for example,Day 1 → Day 3 or Day 5 → Day 7), in the maximum price touched by the stock duringthe 10-day period (
choose the closest amongst the options given)?
On which day is the ratio of the maximum price to the opening price, the highest across the ten days?
For the following questions answer them individually
Wilma, Xavier, Yaska and Zakir are four young friends, who have a passion for integers. One day, each of them selects one integer and writes it on a wall. The writing on the wall shows that Xavier and Zakir picked positive integers, Yaska picked a negative one, while Wilma’s integer is either negative, zero or positive. If their integers are denoted by the first letters of their respective names, the following is true:
$$W^{4}+X^{3}+Y^{2}+Z\leq4$$
$$X^{3}+Z\geq2$$
$$W^{4}+Y^{2}\leq2$$
$$Y^{2}+Z\geq3$$
Given the above, which of these can $$W^{2}+X^{2}+Y^{2}+Z^{2}$$ possibly evaluate to?
If both the sequences x, a1, a2, y and x, b1, b2, z are in A.P. and it is given that $$y > x$$ and $$z < x$$, then which of the following values can $$\left\{\frac{(a1-a2)}{(b1-b2)}\right\}$$ possibly take?
Kim’s wristwatch always shows the correct time, including ‘am’ and ‘pm’. Jim’s watch is identical to Kim’s watch in all aspects except its pace, which is slower than the pace of Kim’s watch. At 12 noon on January 1st, Jim sets his watch to the correct time, but an hour later, it shows 12:57 pm. At 12 noon on the next June 1st, Jim resets his watch to the correct time.
On how many instances between, and including 12 noon on the two dates mentioned, do Jim’s and Kim’s watches show the exact same time, including the ‘am’ and the ‘pm’?
Fatima found that the profit earned by the Bala dosa stall today is a three-digit number. She also noticed that the middle digit is half of the leftmost digit, while the rightmost digit is three times the middle digit. She then randomly interchanged the digits and obtained a different number. This number was more than the original number by 198.
What was the middle digit of the profit amount?
I have five 10-rupee notes, three 20-rupee notes, and two 50-rupee notes in my wallet.
If three notes were taken out randomly and simultaneously, what is the probability that at least 90 rupees were taken out?
The Madhura Fruits Company is packing four types of fruits into boxes. There are 126 oranges, 162 apples, 198 guavas and 306 pears. The fruits must be packed in such a way that a given box must have only one type of fruit and must contain the same number of fruit units as any other box.
What is the minimum number of boxes that must be used?
Consider the real-valued function $$f(x)=\frac{\log{(3x-7)}}{\sqrt{2x^{2}-7x+6}}$$ Find the domain of f(x).
Two Factor Authentication
Incase of any issue contact support@cracku.in